
Trigonometry holds a special place in my heart as it seamlessly bridges the gap between abstract mathematical concepts and their practical applications in the real world. Its importance goes far beyond the boundaries of the classroom.Trigonometry is my favorite subject because it serves as a powerful tool to understand and solve problems involving angles, distances, and relationships between different elements. Trigonometry has a significant impact on how our modern world is shaped, from the planning and constructing of structures to the navigation of spacecraft. It supplies the necessary instruments for measuring heights, distances, and angles, making it a crucial component of disciplines like engineering, physics, astronomy, and even computer graphics.
In this blog post, we'll embark on a journey to unravel the mysteries of trigonometry, starting from the very basics. We'll begin by establishing a solid foundation through essential vocabulary, dive into the significance of trigonometric ratios, explore the utility of standard angles, and finally, equip you with invaluable tips to make proving trigonometric identities an achievable task. Whether you're a student tackling trigonometry for the first time or someone seeking a refresher on these fundamental concepts,this blog post will serve as a beginner's guide to trigonometry. So, let's dive in and unravel the mysteries of this mathematical marvel together.



In our daily lives, when confronted with challenges like calculating the height of an electric pole without a measuring tape, finding the angle a ladder makes with the ground for safety, or determining the distance of a ship on the horizon, trigonometry comes to our rescue.By utilizing angles of elevation, known distances, and trigonometric ratios, we unveil the hidden dimensions of our physical world.Trigonometry transforms complex real-world challenges into solvable equations.

What is Trigonometry?
Trigonometry is a branch of Mathematics which deals with the relationships between angles and sides of a triangles. By utilizing trigonometric Principles and formulas, one can find the inaccessible measurements like we encountered in the real life scenario’s.

In the realm of trigonometry, key terms serve as the backbone, providing the fundamental language for understanding the relationships between angles and sides of triangles. When we delve into the significance of terms like 'angle,' 'hypotenuse,' 'sine,' 'cosine,' 'tangent,' and 'trigonometric identities,' we find that they are not just mathematical abstractions but essential tools for solving real-world problems. From calculating the height of a distant mountain using the concept of angles to navigating an aircraft or ship across the globe, trigonometry's key terms are the guiding stars.
Whether you're a student just beginning your trigonometric journey or a professional applying these principles in your work, these key terms form the very foundation of your endeavors.
Key Terms in Trigonometry:
Angle: A measure of rotation, typically in degrees, radians, or grad, used as a fundamental concept in trigonometry.
Right Triangle: A triangle with one angle measuring 90 degrees, forming the basis for many trigonometric calculations.

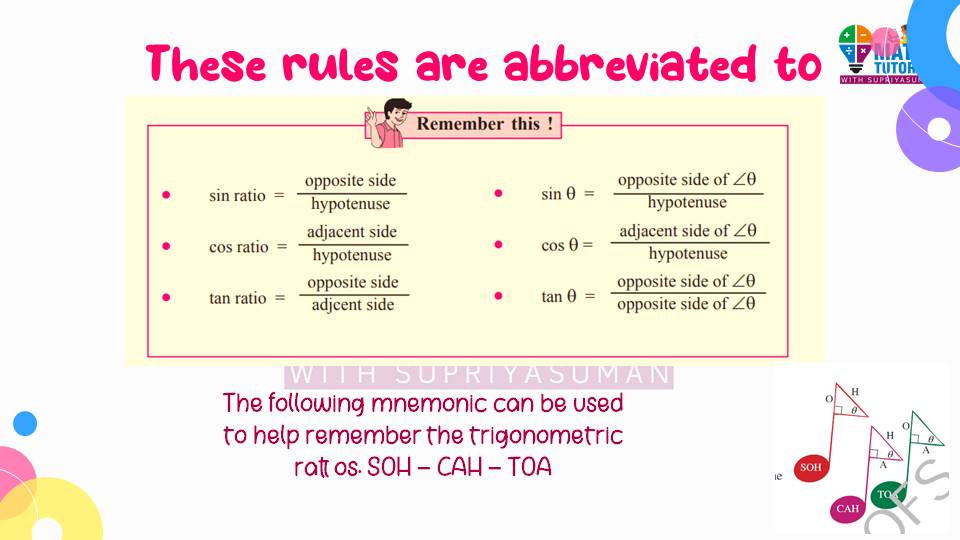
Trigonometric ratios
Trigonometric ratios are the secret codes that unlock the mysteries of triangles and angles. They are the fundamental tools that allow us to establish relationships between the sides and angles of a triangle, paving the way for various practical applications. Sine, cosine, and tangent, along with their reciprocals, cosecant, secant, and cotangent, form the core of these ratios, enabling us to calculate unknown sides or angles in a triangle.In this blog post, we'll unravel the secrets of trigonometric ratios, making them accessible and understandable. We'll explore how these ratios simplify complex problems and enhance our understanding of the world around us.
Trigonometry, often seen as a complex branch of mathematics, becomes more approachable and practical when we understand the concepts of reciprocal and quotient ratios. Reciprocal ratios, like cosecant, secant, and cotangent, are essentially the inverses of the familiar sine, cosine, and tangent. They come into play when we need to find the length of a side or an angle in a right triangle, simplifying complex calculations.
Moreover, quotient ratios, derived from dividing one trigonometric function by another, provide crucial information about the relative relationships between angles and sides in a right triangle. Their utility extends to practical problem-solving scenarios, enabling us to tackle intricate calculations with ease and precision.


In the realm of trigonometry, standard angles hold a special place, serving as guiding beacons that simplify complex calculations and pave the way for a deeper understanding of trigonometric functions. These standardized angles, including 0°, 30°, 45°, 60°, and 90°, offer valuable insights into the fundamental properties of sine, cosine, and tangent functions. Moreover, standard angles play a pivotal role in various practical scenarios, allowing us to effortlessly evaluate trigonometric values and make informed decisions.

Trigonometric Identities: The Crucial Keys to Unlocking Trigonometry's Power

In the world of trigonometry, there's a set of hidden gems known as trigonometric identities, which hold immense significance in simplifying complex mathematical expressions and equations. These identities, including the Pythagorean identity, reciprocal identities, and many more, are not just abstract mathematical concepts but the focal point for practical problem-solving across a spectrum of fields.
Basic Identities and its Variations
At the heart of trigonometry lie the basic trigonometric identities, forming the foundation for comprehending the intricate relationships between angles and sides of triangles.


Mastering Trig Identities: Tips and Tricks for Problem Solving
Trigonometric identities, with their complex-looking equations and intricate relationships, often pose a challenge to students and professionals alike. However, with the right approach and a few clever strategies, tackling problems based on these identities becomes much more manageable.From recognizing fundamental patterns to employing strategic manipulations, understanding these strategies can make a world of difference in unraveling complex trigonometric equations.
One crucial tip is to memorize the core identities and understand their derivations, enabling you to apply them intuitively in various contexts. In this blog post, we'll uncover a treasure trove of tips and tricks, equipping you with the tools you need to confidently approach and conquer problems based on trigonometric identities. Join us on this enlightening journey, as we delve into the art of mastering trig identities and unleash the power of effective problem-solving in the realm of trigonometry.

Separating the left side (LS) from the right side (RS) is indeed a fundamental step in proving trigonometric identities. This helps you focus on simplifying each side individually. If you'd like a step-by-step guide on how to do this, I'd be happy to provide help you on this.

An effective strategy is to start your approach from the more complex side. This method allows you to simplify the problem gradually, making it easier to handle. By beginning with the more intricate xpression, you can apply the identities or manipulations that lead to a simpler form, ultimately reaching the desired solution.

Here's an example on how to approach solving trigonometric identities by beginning with the more complex side:

Solving a trigonometric identity is like navigating a maze; it's crucial to keep your destination in sight. Identify the side of the equation you want to simplify or reach, and let that guide your strategy.Consider the desired outcome at each stage of simplification, making intentional moves toward your target.Fundamental trigonometric identities serve as the building blocks of your solution. Memorize key identities, including Pythagorean identities, reciprocal relations, and quotient relations. These identities provide a toolkit for rewriting trigonometric expressions in equivalent, often simpler, forms. When faced with a complex expression, consider making substitutions using these fundamental identities.

Solving trigonometric identities often requires more than just a deep understanding of trigonometry itself. Integrating various algebraic techniques can provide valuable insights and pave the way to elegant solutions. Let's explore some key strategies:

a) Expand:
Expanding expressions involves opening up brackets and simplifying terms. This technique is particularly useful when dealing with powers of trigonometric functions,sums or differences of terms,multiplying out expressions.By distributing terms and simplifying, you unveil hidden relationships and pave the way for further manipulations.


b) Get a Common Denominator: When confronted with fractions or complex expressions, obtaining a common denominator is a potent technique. This simplifies the process of combining terms and facilitates a unified expression.This technique is particularly effective when dealing with trigonometric fractions, enabling you to unite terms seamlessly.

c) Factorization: Factorization is a powerful tool in simplifying complex expressions.Look for common factors, differences of squares, or sums of squares within the trigonometric expression.This technique often transforms seemingly intricate expressions into more manageable forms.

d) Simplify: Sometimes, the key to conquering a trigonometric identity lies in simplifying both sides of the equation. Use basic algebraic simplification techniques to streamline the expression. Combine like terms, distribute constants, and organize the equation in a way that highlights its essential features.
Incorporating these algebraic techniques alongside trigonometric identities can open up new avenues for problem-solving. Don't hesitate to mix and match strategies—expanding to reveal patterns, getting a common denominator to combine terms, and factorizing to expose hidden relationships. Experimentation and creativity play a significant role in finding the most efficient route to the solution.

Using the conjugate of the denominator is a valuable technique in proving trigonometric identities. This method is particularly helpful when you encounter expressions with square roots, complex fractions, or expressions involving both sine and cosine.Multiply both the numerator and the denominator by the conjugate of the denominator. This means changing the sign between the terms in the denominator.Use the properties of the conjugate to simplify the expression. The goal is often to eliminate square roots or complex fractions.Consider sinx/1+cosx. Multiplying by the conjugate of the denominator 1−cosx, transforms the expression into a more manageable form. This technique streamlines the identity, making it easier to work with and unveil the underlying relationships.Mastering this technique provides a powerful approach to untangling complex trigonometric identities. So, the next time you encounter a trigonometric puzzle, remember the denominator conjugate—a key to unlocking the solution.

Converting expressions into sine and cosine functions is a valuable strategy when proving trigonometric identities. This technique allows you to work with fundamental trigonometric relationships and often simplifies complex expressions.Convert tangent, cotangent, secant, and cosecant functions into combinations of sine and cosine. Utilize the fundamental identities such as tan(x)=sin(x)/cos(x),cot(x)=cos(x)/sin(x) and sec(x)=1/cos(x) to express these functions in terms of sine and cosine.The conversion of expressions into sine and cosine is a transformative strategy in proving trigonometric identities.

In the intricate realm of trigonometric identities, a key tip to mastering the art lies in working each side separately. Begin by isolating one side and meticulously applying trigonometric identities, algebraic manipulations, and simplifications. Let the equation evolve organically. Then, mirror this process on the other side too.The magic happens when, after independent transformations, both sides of the equation converge into the same expression. Achieving this symmetry is a testament to the equality of the two sides.Embrace this tip, work with precision, and witness the satisfying moment when both sides of the identity stand united in equality. Happy proving!
Conclusion
As we bring the curtain down on our exploration of "Trigonomagic: Mastering Tips and Tricks for Proving Trigonometric Identities with Ease!"Let's take a moment to appreciate the adventure we've had. We kicked things off by getting to know the basics of trigonometry, understanding its ins and outs and then delving into the important world of basic identities.
But the real excitement came when we started talking about the cool tips and tricks that make proving trigonometric identities much easier. These aren't just academic tricks; they're like secret codes that have helped many of my Trigonometry students not only understand trigonometry better but also get awesome grades in the subject.
It's super cool to hear about students who used to find trigonometry tricky but are now acing their tests thanks to these simple strategies. These tricks are not just about getting good grades; they're about giving students the confidence to tackle math challenges .May these tips continue to be helpful, lighting up the path to success for many more students who are navigating the world of trigonometric identities.
Here's to making trigonometry feel more like magic and less like a puzzle! Happy proving!Those who are looking to learn any topic of Prealgebra,Algebra 1,2,Geometry,Trigonomety . Happy Learning.



Comments